We could carry out such integrals for all sorts of different shapes, although many of them are inetgrals over areas or volumes instead of over lengths. X 3] with upper limit L and lower limit 0įor a uniform rod rotating about one end. Split the rod into little pieces of size dx. How do we evaluate the moment of inertia integral:įor a uniform rod of length L rotating about an axis passing through one end of the rod, perpendicular to the rod?Īlign the rod with the x axis so it extends from 0 to L.
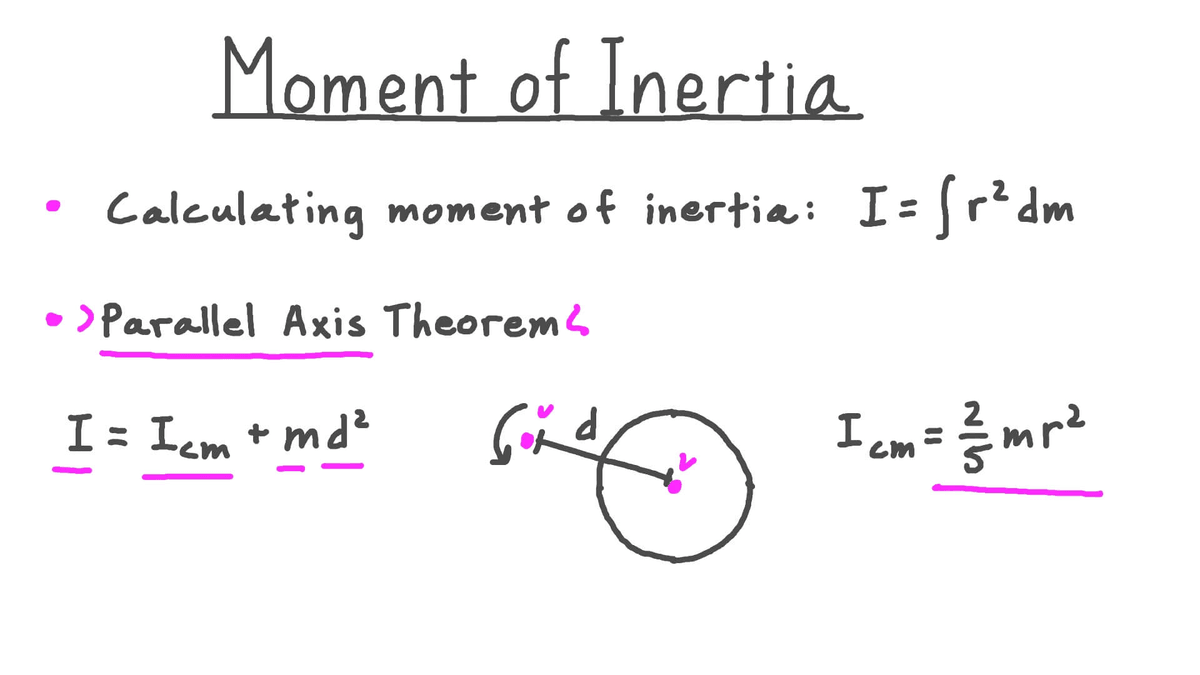
The moment of inertia, I, is the rotational equivalent of mass.įor a simple object like a ball on a string being whirled in a circle, where all the mass can be considered to be the same distance away from the axis of rotation, the moment of inertia is:įor something more complicated, where mass is distributed at different distances from the rotation axis, the moment of inertia is determined by integrating:Įxample - a uniform rod of length L rotating about one end